【精采推理】+【幽默對白】+【中英雙語讀本】+【英語有聲小說】
臺灣首部展現亞洲風土民情的雙語推理小說,
貼近生活,學習可在日常中使用的口語英文!
★新加坡國際學校圖書館紅點圖書大賞續作★
★全系列屢獲新加坡讀者票選好書獎★
★全球售出中、美、韓等八國版權★
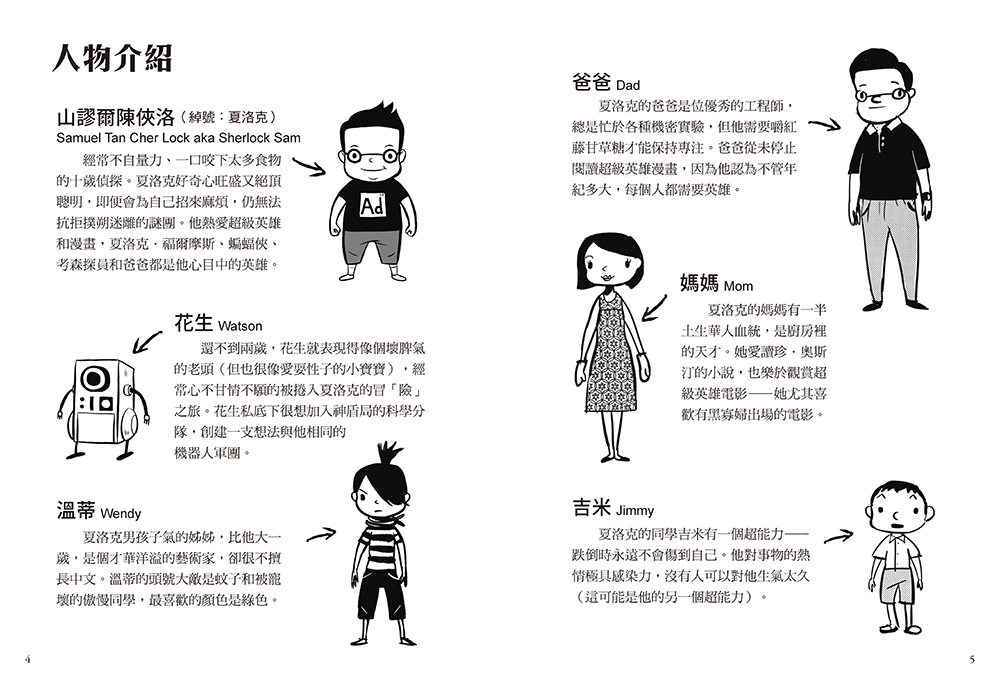
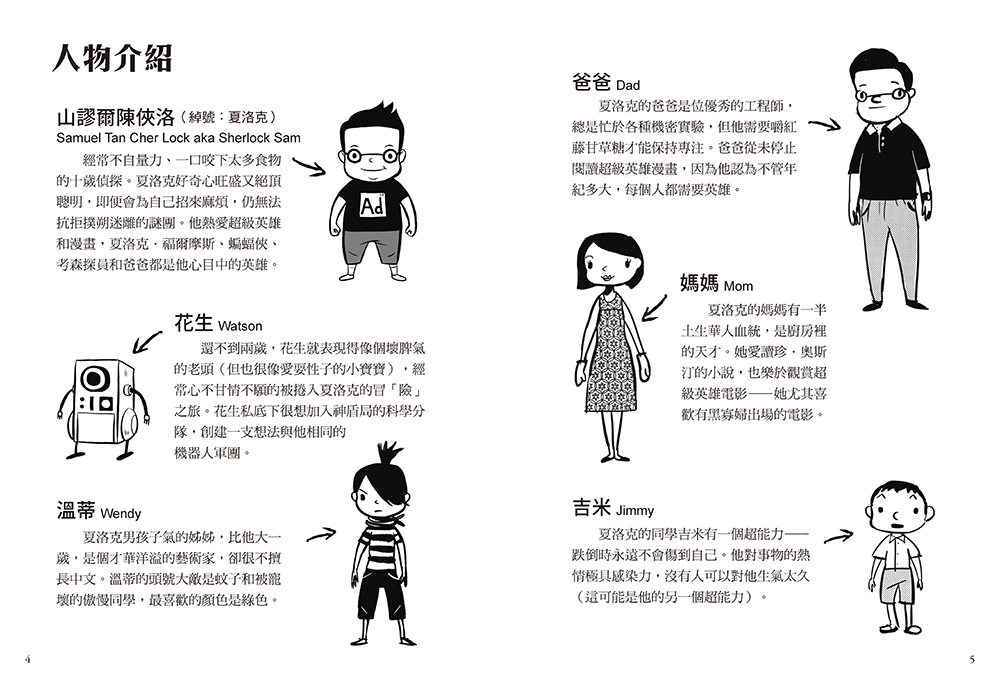
好不容易解決「不翼而飛的機密劇本」案,夏洛克以為自己阻止了邪惡主謀的詭計,卻震驚發現他的最佳拍檔──花生,竟被邪惡主謀綁架,下落不明!邪惡主謀指示要夏洛克參與他的「遊戲」,否則就再也見不到花生,夏洛克只好遵循邪惡主謀提供的線索,一步步解開謎題。
他們破解字謎、找出數列的規律、憑藉陌生的中文字搜索地標、找出藏在化學元素列表中的祕密……儘管破解一道道謎題,夏洛克卻覺得他們被玩弄於股掌之間,離花生的所在地越來越遠。
幸好在心亂如麻的夏洛克身邊,有堅定可靠的爸爸媽媽與宵夜幫夥伴相伴。然而,夏洛克卻遲遲無法接納曾與邪惡主謀來往的叛徒。他牢牢築起的心房與猜疑的眼光,能保護剩下的宵夜幫不受傷害嗎?還是反倒會使夥伴關係分崩離析?
為了拯救親愛的家人,夏洛克用盡全力,但他是否還剩下那麼一點點勇氣,能接納痛改前非的往日好友,攜手帶回落入敵手的花生,並探尋邪惡主謀真正的目的?
「夏洛克與花生」系列──「邪惡主謀的挑戰書」篇,完美落幕!
「夏洛克與花生」系列為臺灣第一部描繪亞洲風土民情的雙語偵探小說。故事背景新加坡的國民大多是華人,有著與臺灣相近的生活習慣、家庭關係與升學環境,卻說著一口流利的英語。閱讀本系列小說,即可學會在小吃店點餐、介紹特產與景點等可在生活中運用的口語英文。
本系列採「中英雙語」編輯,前半部為中文翻譯、後半部為英文原文。讀者讀完中文小說,了解劇情後再用英文讀一遍,更能掌握英文的用詞、表述方式。中文加注文化注釋,有助理解小說中描繪的東南亞文化;英文則加注單字、片語釋義,並延伸補充英文應用知識,無須經常查辭典,便可更專心享受閱讀的趣味。掃描書上的QR Code下載英語有聲書音檔,還可用耳朵「聽」小說,不僅打發通勤、運動等零碎時間,亦可同步增進英聽能力。
全書最末有三個補充專欄。「口說練習」從英文小說中挑選六句日常生活經常使用的實用句子,並提供專業錄製的口說練習音檔,多聽多說,練就可自然運用道地英語的表達能力;「單字測驗」提供六題英文克漏字測驗,讀完小說小試身手,測試自己學會多少單字,加深對生詞的印象。「敘述人物」以英文短文描述宵夜幫成員,將正確敘述配對到對應的角色身上,考驗單字量與短文閱讀能力!學英語就是要眼耳口手齊動員,別再只是死記硬背!
加入夏洛克與「宵夜幫」的精采冒險,
聽.讀.說.寫學英文!
動腦破解邪惡主謀的謎語,救出被綁架的花生!
★令人嘴角上揚的幽默推理故事,在歡笑中愛上英語聽.讀.說.寫!
聽:掃描QR Code,有聲書帶著走;隨時隨地輕鬆聽,鍛鍊聽力不費勁。
讀:加注單字、片語解釋,補充英文應用知識,減少查單字,專心讀故事!
說:聲調仔細聽,開口勤練習;實用短句音檔,帶你說出流利英語。
寫:讀完英文小說後,來場克漏字測試。檢視你習得多少單字!
★「夏洛克與花生」系列(第一季全八冊上市!)
‧《夏洛克與花生2:歷史公園的鬼吟聲之謎》
‧《夏洛克與花生3:國際學校的恐嚇信之謎》
‧《夏洛克與花生4:外星人入侵烏敏島之謎》
‧《夏洛克與花生5:博物館機器人盜竊之謎》
‧《夏洛克與花生6:天才少年憑空消失之謎》【邪惡主謀的挑戰書〈上〉】
‧《夏洛克與花生7:機密劇本不翼而飛之謎》【邪惡主謀的挑戰書〈中〉】
‧《夏洛克與花生8:最佳拍檔花生綁票之謎》【邪惡主謀的挑戰書〈下〉】
A.J. Low
A.J. Low 是由一對夫妻組成的寫作團隊。
丈夫阿丹.希門尼斯除了是名作家,也是翻譯與編輯。他出生於美國,是一對墨西哥移民夫妻引以為傲的兒子;從美國搬到新加坡後,他自己也成為移民。他喜歡漫畫、樂高、書(尤其是科幻小說)、遊戲和美食。
妻子費莉西雅.劉 - 希門尼斯在新加坡出生長大,曾是一位書商,目前從事漫畫出版工作。她有空時不是在閱讀、打盹,就是循環播放音樂。
黃筱茵
臺灣師範大學英語研究所博士班(文學組)學分修畢。曾任編輯,翻譯超過三百冊繪本與青少年小說。譯作有「亞瑟王」系列、《許願樹》、《彼得與他的寶貝》等。
編輯序
人物介紹
第一章:家人的回憶
第二章:惡魔的遊戲
第三章:第一道關卡
第四章:解密數字
第五章:中文的考驗
第六章:扳回一城
第七章:對決邪惡主謀
第八章:花生的遭遇
第九章:倒楣的原因
第十章:主謀的自白
英文小說注釋符號說明
導讀
Chapter One
Chapter Two
Chapter Three
Chapter Four
Chapter Five
Chapter Six
Chapter Seven
Chapter Eight
Chapter Nine
Chapter Ten
口說練習Speak English with Sherlock Sam
單字測驗Vocabulary Challenge
敘述人物 Meet the Supper Club!
第四章
我們走下樹屋剩餘的臺階。這數列讓我感覺似曾相識,但我想不出是為什麼。
「還有誰覺得這些數字似曾相識嗎?」我問,坐在最底下的臺階。吉米坐在我旁邊,其他人則圍成半圓形站著。
除了爸爸和媽媽以外,大家都搖頭。「沒錯,確實感覺似曾相識。」爸爸說:「不過我不曉得為什麼。」
「我也是。」媽媽說:「但沒錯,確實很熟悉。」
「那些不是《LOST 檔案》 裡面的數字,對吧?」溫蒂說道,我搖搖頭。
「說不定那是座標?」納札爾說:「像經度和緯度?」
「我不這麼覺得,因為經緯度通常是以度、分、秒來標示的。」我說:「這串數字太長了。」
「也許第四個數字是秒的小數?」伊萊莎問道。
「伊萊莎,我不這麼覺得。」我說,沒有看她。
「但檢查一下也不會怎麼樣,對吧?俠洛?」溫蒂說。我瞪著她搖搖頭,不過她也不是在徵求我的同意。
伊萊莎拿出手機,輸入緯度二十一度十三分八點五秒,經度三度兩分一點一秒。
她吐出一口氣,「除非詹姆斯能在放學後到現在的這段時間內,飛到阿爾及利亞南部的沙漠,否則它們就不是經緯度座標。」
「納札爾,這有可能是日期嗎?」溫蒂問。納札爾是我們的常駐歷史專家,所以若有任何人能回答這個問題,絕對就是他了。
「也許,但可能不是使用公曆。」納札爾解釋:「如果我們把數字分成兩部分,可以解讀成西元八○五年第十三個月第二十一天,或西元二一一三年第八個月第五天。但公曆沒有第十三個月,而詹姆斯也不可能預知未來。」
「其他的曆法呢?」爸爸問:「像猶太或伊斯蘭曆?」
「這個嘛,就我所知,沒有任何曆法有十二個以上的月分,但有些曆法早在公曆出現的很久以前就開始流傳,所以二一一三年實際上可能指的是過去,而不是未來。」納札爾說:「舉個例子,希伯來曆就會說西元二○○○年實際上是五七六○年。」
「那就表示希伯來曆的二一一三年相當於公曆的西元前一六四九年。」我說。
「那個時候發生了什麼事?」吉米問:「有一場大戰還是什麼嗎?」
納札爾聳聳肩,「我的歷史知識並不完美。」
「據網路所說,埃及的中王國時期結束了。」爸爸說。
「會不會是不同的曆法系統呢?」我問:「或是不同的座標系統?」我滿懷希望的抬頭看向爸爸媽媽。
「山姆,我不這麼認為。」爸爸說:「要是我們能弄清楚它們為什麼感覺這麼熟悉就好了……」
我真的被難倒了,對這些數字可能代表什麼毫無頭緒。
其他人好像也一樣。我開始害怕我們永遠也無法找到花生,尤其是我們才到第二關就卡住了。
「說不定跟數學有關?」伊萊莎終於開口說:「所以你們才全都覺得很熟悉?」
「或許吧!」我說:「但是有這麼多不同的數字系統、方程式與常數,我實在不曉得這些數字會對應什麼。」
「數字在遞減,對吧?」她說:「有變小的模式嗎?」
我再次看向這些數字,精神為之一振,「對吔!確實有模式。是單純的減法模式。把第一個數字減掉第二個數字,就得到第三個數字。這個數列裡任三個連續數字都是這樣,所以二十一減十三等於八,十三減八等於五,以此類推。」
「但那還是沒有真正告訴我們任何事呀!」媽媽說。
就在這時,爸爸大喊:「我懂了!這是斐波那契數列!我不敢相信我竟然沒有馬上看出來!」
我盯著那些數字:21、13、8、5、3、2、1、1。爸爸說得沒錯!是斐波那契數列,只是順序倒過來而已!我確信如果我們沒有這麼擔心花生,爸爸和我早就注意到了。
「為什麼要破壞納奇?」吉米皺著鼻子問。
「什麼?破壞?什麼?」溫蒂問,她看起來非常困惑。
「麥可叔叔說『非破納奇』啊?」
「是斐波那契,吉米。」我說:「他是一位義大利數學家,印度-阿拉伯數字系統就是在他的幫助下傳遍歐洲,成為當今全世界最普及的數字系統。斐波那契數列有助於描述自然界中的許多螺旋,包括DNA 螺旋、花瓣分枝、颶風,甚至是銀河的螺旋等。」
「好,所以那是倒過來的斐波那契數列,」媽媽說道:「那對我們有什麼幫助呢?」
我們的興奮之情消逝得和它出現時一樣突然。我們現在知道那串數列是什麼了,卻不知道它該引領我們走向何方。像我剛才說的,斐波那契數列在大自然中隨處可見,意味著它能出現在宇宙的任何地方。事實上,我敢說一旦我們找到多重宇宙存在的證據,我們就會發現無限的宇宙以螺旋狀排列,並完全符合斐波那契數列。
「嘿!那科學中心外面的臺階呢?」吉米說:「根據剛才的標示,它們是『非破納奇』臺階,對不對?」
Chapter Four
We walked down the rest of the steps of the tree house. The number sequence seemed familiar to me, but I couldn’t figure out why.
“Do these numbers seem familiar to anyone else?” I asked, sitting on the bottom step. Jimmy sat next to me, while the rest stood around in a semicircle.
Everyone shook their heads except Mom and Dad. “Yes, it does seem familiar,” Dad said, “but I don’t know why.”
“Me either,” Mom said, ”but yes, really familiar.”
“They’re not the Lost numbers, right?” Wendy said. I shook my head.
“Maybe they’re coordinates?” Nazhar said. “Like latitude and longitude?”
“I don’t think so, as that’s usually measured in degrees, minutes and seconds,” I said. “There are too many numbers here.”
“Maybe the fourth numbers are the decimal places after the seconds?” Eliza asked.
“I don’t think so, Eliza,” I said, not looking at her.
“But it wouldn’t hurt to check, right, Cher Lock?” Wendy said. I glared at her and shook my head, not that she had been asking for my permission.
Eliza took out her phone and entered the numbers as 21 degrees, 13 minutes and 8.5 seconds latitude, and 3 degrees, 2 minutes and 1.1 seconds longitude.
She let out a puff of air. “Unless James was able to fly to the deserts of southern Algeria between now and when school let out, they’re not latitudinal and longitudinal coordinates.”
“Could they be dates, Nazhar?” Wendy asked.
Nazhar was our resident history expert, so if anyone would know, it would be him.
“Maybe, but probably not using the Gregorian calendar,” Nazhar explained. “If we take half the numbers, that would mean the first four numbers can be read either as the 21st day, 13th month of the year 0805, or as the year 2113, 8th month and 5th day. However, there’s no 13th month in the Gregorian calendar and there’s no way James can predict the future.”
“What about other calendar systems?” Dad asked. “Like the Jewish or Islamic calendars?”
“Well, as far as I know, no calendar has more than 12 months, but some calendars start much earlier than the Gregorian calendar, so the year 2113 would actually be in the past and not the future,” Nazhar said. “For example, the Hebrew calendar would say that the year 2000 is actually the year 5760.”
“That would make 2113 on the Hebrew calendar equivalent to the year 1649 BCE on the Gregorian,” I said.
“What happened then?” Jimmy asked. “Was there a great battle or something?”
Nazhar shrugged his shoulders. “My knowledge of history isn’t perfect.”
“The Middle Kingdom ended in Egypt, according to the internet,” Dad said.
“Could it be a different calendar system?” I asked. “Or a different coordinate system?” I looked up at Mom and Dad hopefully.
“I don’t think so, Sam,” Dad said. “If we could just figure out why they seem so familiar…”
I was really stumped, and had no idea what these numbers could mean. And it seemed everybody else was too. I was starting to fear we would never find Watson, especially if we were stuck on only the second riddle.
“Maybe it’s a maths thing?” Eliza finally said. “That’s why it seems familiar to all of you?”
“Maybe,” I said, “but there are so many mathematical number systems, equations and constants that I have no idea which one these numbers would correspond to.”
“Well, they’re decreasing, right?” she said. “Is there a pattern in how they’re getting smaller?”
I looked at the numbers again and I perked up. “Yeah, there is a pattern. It’s a simple subtraction pattern. You subtract the second number from the first, and you get the third. And this works with any three consecutive numbers in series, so that 21 minus 13 equals 8, and 13 minus 8 equals 5, and so on.”
“But that still doesn’t really tell us anything,” Mom said.
Just then, Dad shouted, “I got it! It’s Fibonacci! I can’t believe I didn’t get it right away!”
I stared at the numbers: 21 13 8 5 3 2 1 1. Dad was right! It was the Fibonacci sequence, but backwards! I was sure if we hadn’t been so worried about Watson, Dad and I would have noticed that sooner.
“Why would lying to pasta help?” Jimmy asked, wrinkling his nose.
“What? Pasta? What?” Wendy said, looking confused.
“Uncle Mike said ‘fib-a-gnocchi’?”
“Fibonacci, Jimmy,” I said. “He was an Italian mathematician who helped spread the Hindu-Arabic numeral system throughout Europe, and it is now the most common numeral system in the world. The Fibonacci sequence helps to describe many spirals found in nature, including DNA spirals, petal branchings, hurricanes and even galactic spirals.”
“Okay, so it’s the backwards Fibonacci sequence,” Mom said. “How does that help us?”
Our excitement disappeared as suddenly as it had appeared. We knew what the number sequence was now, but we didn’t know where it was supposed to lead us. As I had said, the Fibonacci sequence occurred all over nature, and that could literally mean anywhere in the universe. In fact, I was sure that once proof of the multiverse was discovered, we would find that the infinite universes were arrayed in spirals conforming to the Fibonacci sequence.
“Hey! What about the steps outside the Science Centre?” Jimmy said. “According to the sign just now, they’re fib-a-gnocchi steps, right?”